A fixed point for black hole distributions

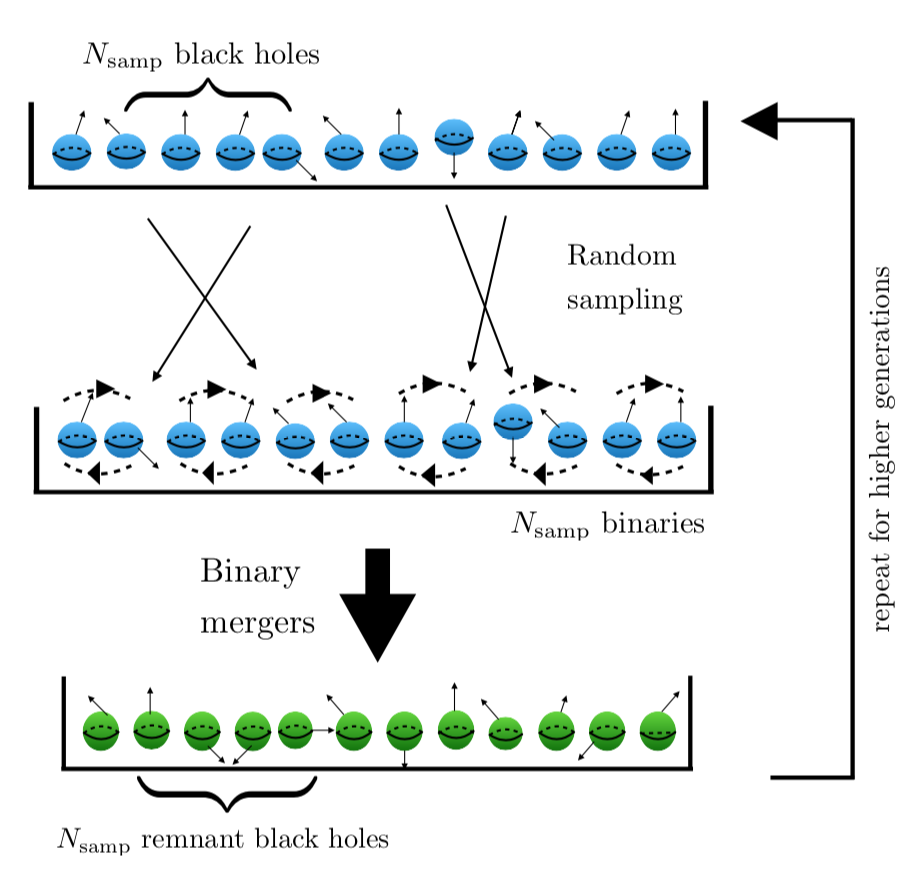
Understanding distributions of black holes is crucial to both astrophysics and quantum gravity. Studying astrophysical population statistics has even been suggested as a channel to constrain black hole formation from the quantum vacuum. Here we propose a Gedankenexperiment to show that the non-linear properties of binary mergers (simulated with accurate surrogate models) generate an attractor in the space of distributions. Our results show that the joint distribution of spin magnitude and fractional mass loss evolves to a fixed point, converging in a few generations. The features of this fixed point distribution do not depend on the choice of initial distribution. Since a black hole merger is irreversible it produces entropy – possibly the largest source of entropy in the universe. The fixed-point distributions are neither isothermal nor isentropic, and initially thermodynamic states evolve away from thermality. We finally evaluate the specific entropy production rate per merger from initially thermal and non-thermal distributions, which converges to a constant.